Ever seen girl math?
“If I preload my Starbucks account with $25 and I go to Starbucks the following week, my order was free.”
“Spending money abroad doesn’t count because it’s a different currency.”
Things aren’t mathing as they should.
As I recently learned,
“If I return clothes to the store (store credit), but then buy new clothes (using that store credit), those new clothes are free. (No new money spent)”
They aren’t free but they are a sunk cost so you might as well
ah yeah girl math where
“common irrational human psychology” + “deepseated hatred of women” = whatever the fuck this take is
What makes this “girl” math?
Anecdotally, I’ve only heard women use that sort of argument IRL. Kinda falls in the, “This widget is 80% so I’m saving money!”
Well, no, not if you didn’t have to purchase the widget in the first place.
Men are hardly immune, but in my experience it’s more so women.
Sexism 👉👉
Stupid people standing on soapboxes saying stupid shit.
Back in my day, people had to dedicate years of their life before they were given the opportunity to stand in front of hundreds of people and tell them things.
Brutha - they call it standing on a soapbox for a reason. And soapboxes havent physically been a thing for a real long time. Wait’ll you bear about “stumping”.
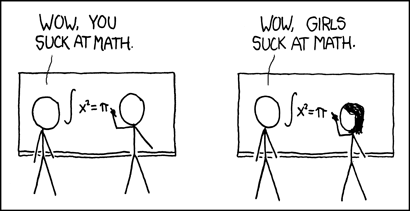
The different ways in which numbers slide up, down, sideways, diagonally.
Is the example in the post part of the fifth type of arithmetic?
- Addition +
- Subtraction -
- Multiplication x
- Division /
- Modulo %
The first time I learned about modulo as its’ own branch of arithmetic was long out of school already, I had only hazily heard of it, on a PBS Nova documentary in the 1990s about Fermat’s famous theorem and when it was proven after centuries of failed tries.
No it’s not modulo, it’s how to talk about increasing a number by a percentage of the number.
Yeah we just learned “by” was a standard term for multiplication. So increased by 80% was just 1.8 times whatever you started with. “Divide by” meaning multiply inversely
Language translating to artithmetic. I’m sure it doesn’t always line up, as we change language quite often.
I think it’s ambiguous and the 90% actually makes more sense. If you increase something by 5m you are taking the original value and adding 5m to it. For multiplication you should probably avoid the word increase and say scaled by instead. 10% scaled by 180% is 18%.
Even more confusing when you hear that the odds of catching a disease have increased by a %. In many ways odds can be more intuitive, but we’re so used to working with simple probability that it’s a total nightmare to wrap your head around at first.
well it’s ambiguous. Its also a sloppy way of expressing an increase by 80 percentage points.
That’s not sloppy, that’s simply wrong
Fair enough, I’m inclined to agree. It’s a relatively common error though, still leaving it ambiguous outside of circles where you expect people to express themselves with mathematical precision.
hopeful-weirdo just needs to be told to consider what increasing by 500% means and it’ll click.
Dark Souls cleared this up for me real quick.
Funny thing is this is a language issue, not a math issue.
Why not both?
I’ve always thought of math as a language and I talk to my kids about it that way too. Math is an other way to describe the world.
It’s very different from spoken languages and translating between the two needs to be learned and practiced.
Our math education doesn’t include enough word problems and it should be bi-directional. In addition to teaching students how to write equations based of sentences we should teach them how to describe what’s going on in an equation.
Yeah, it is kinda both in general. Though in this case, the math about this is well-defined: it’s possible to increase a percentage either with addition or multiplication and both of those can make sense, just the words we would use to describe them are the same so it ends up ambiguous when you try going from math to English or vice versa.
But the fact that switching between communication language and a formal language/system like math isn’t clear cut does throw a bit of a wrench in the “math doesn’t lie”. It’s pretty well-established that statistics can be made to imply many different things, even contradictory things, depending on how they are measured and communicated.
This can apply to science more generally, too, because the scientific process depends on hypotheses expressed in communication language, experiments that rely on interpretation of the hypothesis, and conclusions that add another layer of interpretation on the whole thing. Science doesn’t lie but humans can make mistakes when trying to do science. And it’s also pretty well established that science media can often claim things that even the scientists it’s trying to report on will disagree strongly with.
Though I will clarify that the “both” part is just on the translation. Formal systems like math are intended to be explicit about what they say. If you prove something in math, it’s as true as anything else is in that system, assuming you didn’t make a mistake in the proof.
Though even in a formal system, not everything that is true is provable, and it is still possible to express paradoxes (though I’d be surprised if it was possible to prove a paradox… And it would break the system if you could).
Yes. I really think that the translation part is one of the hardest.
As a brief aside, I want to note that this conversation is happening in one of the languages we’re discussing and that could influence any conclusion we come to. I’m also going to suggest that we ignore Gödel for now
There are many people who are good at math. There are even a lot of people who are reasonably good at grinding through the mechanics of math. That doesn’t solve any of the problems you described above.
Statistics are a great example of this. Early statistics classics are mostly about the mechanics; here’s how you calculate the mean, standard deviation, confidence intervals, etc. 2 types of students generally come out of that class; math students who will forget all of that because they’re going to learn the “real” versions (eg they go through a huge number of proofs that involve calculus and linear algebra), and students who will forget all of that because the whole thing sounds like gibberish.
We teach natural languages the same way but we go much farther. Students learn vocabulary and grammar rules but they’re also expected to learn how to use them correctly. We had students current events articles and ask them to analyze them. We ask students to practice many writing methods including fiction and expository writing.
When I talk to my own kids about statistics I never write any formulas. I ask questions like, “What do you think ‘mean’ means?”, “If I have a bunch of <example item> does ‘mean’ describe it well?”, “What happens if I add an <example item> with <huge outlier>? Do you still think it’s a good description?” “How would you describe it better?”
If I ever had to design an introductory statistics course it would contain very little “math”. Classes, homework, projects, and tests would consist of questions like; “Here’s some data and an interpretation, are they lying? Why or why not?” “Here’s a (simple) data scenario. Tell me what’s going on.” “Here’s some (simple) data. Produce a correct and faithful summary. Now produce a correct but misleading summary. Describe what you did and the effect.” “Here’s a conclusion. Provide sample data that most likely fits the conclusion.” “Change one word in the sentence, ‘Increase your chances by 80% means that there is now an 80% chance.’ to make it a true statement.”
Can’t believe nobody has linked the relevant xkcd yet

We appreciate your service.
🫡
🫡
Which of course is why people referred to points when discussing stocks/markets. Got to love an unambiguous term.
People got this wrong about inflation as well. In 2020 there was actual deflation, and in 2021 there was very minimal inflation, meaning prices were still largely lower or similar as 2019. Then we saw 9% inflation in 2022. Total inflation in 2024 vs the 2019 benchmark was around 15%. Or 3% average per year, which is barely over the baseline. People just hear 9% inflation, completely missing the fact that this was a YoY number relative to the Trump recession.
And then there was that bogus article that said Argentina had lowered it’s inflation to 2% and you find out in the article that’s monthly inflation and the yearly figure was like 190%.
If they managed to decrease from 190%/year to 2%/month (which is 27%/year) that’s still an impressive result. Not as impressive to publish when you want to make a click bait.
I’m not sure how to put that to percentages thought, is it 86% and 143 percent points decrease?
Drag doesn’t know exactly what the problem is, but the official inflation figures cannot be right. Housing is so much more expensive. Food is more expensive. And it’s not 9% more expensive. Drag knows they say the math takes into account the price of rent, but they’ve gotta be lying somehow. It’s impossible that the cost of living is rising so much faster than inflation. Those should be the same. If they’re not the same, someone’s math is wrong.
I keep track of my grocery bills going on 10 years now and 14-15% is spot on for what I buy.
Housing is more than 15% more expensive
It may as well be accounted in the inflation, but with a lower weight. Usually the institution responsible for calculation of the inflation will publish the methodology so one can see for emselve, real perceived inflation may be higher or even lower depending on what your consumption profile is.
Won’t your consumption profile necessarily change if rent is raised and you have to buy fewer luxuries? Do the calculations take that into account?
Did this turn into an /iamverysmart thread ?
So you’re telling me there’s a chance?
In the same vein, if the volume on your phone is on 1, and you increase it to 2, it has increased by 100%
i think
Kinda. Logarithms are weird
Why lying with maths is so easy, the average person, even in developed countries is practically innumerate (massive hyperbole, but the fact lying with numbers is easy, still stands)
Difference between increase of x% (old percentage + old percentage * x%)% and increase of x percentage points (old percentage and x)%